Have you ever wondered why some notes sound the same even though they have different names? These notes are called enharmonic equivalents, which occur when two notes have the same pitch but are spelled differently. The sounds of these notes are indistinguishable, but in music theory, they can be used to describe the function of a note in a chord.
The origins of enharmonic notes can be traced back to Ancient Greece, where they were used in tuning instruments. The concept was later developed by medieval theorists and has been used in Western music since the Renaissance.
What Are Enharmonic Notes in Music?
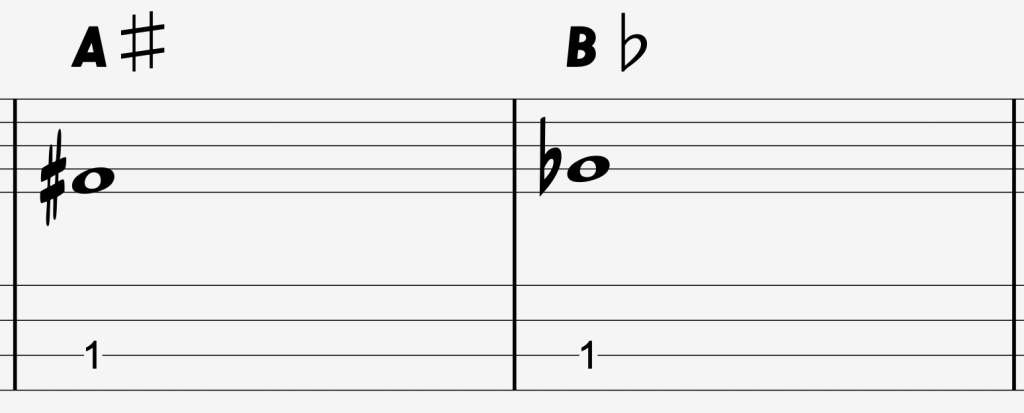
Enharmonic notes are two notes that have the same pitch but are spelled differently. These notes are usually played on different instruments or have different names. For example, the note A♯ (A-sharp) is the enharmonic equivalent of B♭ (B-flat).
Enharmonic notes are often used to describe the function of a note in a chord. For example, note C♯ can be used as the root of a C♯ major chord, or it can be used as the third of an A major chord.
Another way to think of it is looking at the keys on a piano. A C sharp and D flat is the exact same black key on a keyboard. Two different names, same pitch.
Enharmonic Notes Table – Learn the Enharmonic Equivalent for Each Note
The following table shows enharmonically equivalent notes starting with the note A. If you see a “♭♭” that means a double flat. In other words you would lower the pitch by two half steps. You could also see double sharps in the field. Once you see the table below, you’ll get the hang of it quickly and know which spelling works best for you.
Note: While a certain enharmonic spelling may make the most sense from a theoretical perspective, sometimes the most simple spelling is easier to access depending on the musical context.
Table of Notes And Their Enharmonic Equivalents
| Note | Enharmonic Equivalent |
|---|---|
| A | B♭♭ |
| A♯ | B♭ |
| B | C♭ |
| B♯ | C |
| C | D♭♭ |
| C♯ | D♭ |
| D | E♭♭ |
| D♯ | E♭ |
| E | F♭ |
| E♯ | F |
| F | G♭♭ |
| F♯ | G♭ |
| G | A♭♭ |
| G♯ | A♭ |
Enharmonic Equivalents Simplify Music Theory
Why Use Enharmonic Equivalents?
In music theory, enharmonic equivalents are notes that sound exactly the same but are written differently, such as F# and Gb.
This practice helps organize music and makes it easier to understand. Here’s how:
1. Avoiding Redundancy in Key Signatures
When composing in a specific key, it helps that each note name appears only once. For instance, in the key of G major, the scale includes the notes G-A-B-C-D-E-F#-G. Naming the seventh note as F# instead of Gb maintains clarity. If it were named Gb, there would be two types of G (G and Gb), which can create confusion, disorganization, and take longer to process in the mind.
2. Enhancing Readability
Using enharmonic equivalents helps prevent redundancy and keeps sheet music easy-to-read. Musicians can quickly identify notes based on their position within the key signature, without having to think so hard to interpret multiple forms of the same note.
3. Maintaining Harmonic Integrity
Enharmonic equivalents ensure that each note serves its proper harmonic function. In G major, an F# naturally leads to G because of its position in the scale. Naming it Gb would obscure this relationship, complicating the harmonic understanding.
4. Simplifying Modulation
When transitioning between keys (modulating), enharmonic equivalents make the process smoother. For example, transitioning from G major to D major involves recognizing F# in both scales. Using consistent note names makes it easy to recognize common tones and shifts in harmony.
Practical Implications
- For Composers: Ensuring that each note name appears only once within a key helps in maintaining the logical flow of the composition.
- For Performers: Clear notation reduces the chances of misunderstanding and misplaying, thus improving overall performance.
Which Enharmonic Spelling (Sharp or Flat) Should You Use?
There’s a couple school’s of thought when it comes to which enharmonic spelling you should use.
Theoretically, the correct enharmonic spelling is based on the function of the note. For example, a G♭ in the key of D♭ is referring to some sort of fourth. But, a F♯ in the key of D♭ is referring to some sort of third.
On the flip-side, the other school of thought is to use whichever enharmonic spelling is more accessible to your mind. If it’s simply easier and faster to think of D♭ than it is to think of C♯, then use D♭. At the end of the day, the notes sound exactly the same. So, try not to get caught up on spelling.
Adding to that, sometimes it’s simpler to think of a note as a sharp rather than a flat, or the other way around, depending on the direction you are moving in a scale or melody. This can help you navigate scales and melodies more easily, especially during fast-paced playing or sight-reading.
Ultimately, the important thing is to choose the notation that makes your musical journey smoother and more intuitive, leading to a more fluid performance. Whether you prefer D♭ or C♯, what matters most is finding what works best for you.
If you really want to streamline your thought process, check out the Number System in music. A lot of jazz musicians think more in numbers than with note spellings.
The Number System in music is an efficient approach, because you no longer have to deal with enharmonic spellings.








